Amplitudes of Tetrahedral Spin Network

after Dan Christensen and Igor Khavkine;
using the following equations,

The amplitude is the real part of f(q)/g(q),
where q is a complex number,
f(q) = (q^4-q^2+1) (q^4+q^3+q^2+q^+1) (q^4-q^3+q^2-q^+1) (q^6+q^5+q^4+q^3+q^2+q^+1) (q^6-q^5+q^4-q^3+q^2-q^+1) (q^20-q^18-q^14-q^12+q^10-q^8-q^6-q^2+1)
and
g(q) = (q^8)((q^4+q^2+1)^2) ((q^4+1)^5).

The value of amplitude is colored using the modulation function,
adjust(x) = log(log(abs(x)+1)+1).



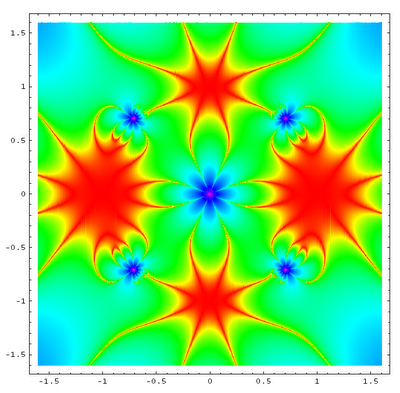
The argument of the result using the same modulation function for the color description.


Recover the negative (-Pi, 0) and positive (0, Pi) difference in the argument.


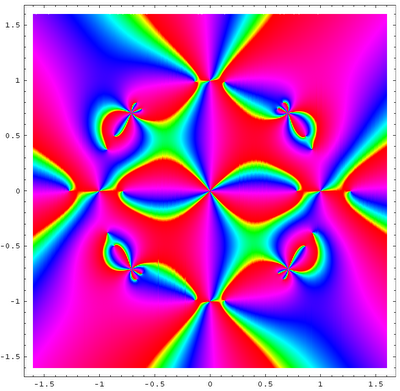
still not understand what it means..